|
Réponse |
Message 1 de 36 de ce thème |
|
Ancient cultures knew about sacred geometry reflected in nature and derived their individual measures of length from it. Many ancient writings suggest that this knowledge was given to mankind by god(s). The ‘Gods’ of certain cultures could be early post-flood founders a few generations after Noah. In Egypt, building overseers required the Royal Egyptian Cubit to be calibrated against a precision standard at regular intervals. Failure to do so was punishable by death. This extreme respect for the royal cubit indicates an important legacy, like a standard handed down from the ‘Gods’.
According to the “Secrets of the Great Pyramid” (by L. Stecchini) the Egyptian measures of length, originating from at least the 3rd millennium BC, were directly derived from the circumference of the earth with an amazing accuracy. On page 346, his claim is that the Egyptian measurement was equal to 40,075,000 meters, which compared to the International Spheroid of 40,076,596 meters gives an error of 0.004%. No consideration seems to be made to the question of, on purely technical and procedural grounds, how the early Egyptians, in defining their cubit, could have achieved a degree of accuracy that to our current knowledge can only be achieved with very sophisticated equipment and techniques.
Note: Egyptians calculated polar radius as 12,000,000 Royal Cubits (of 0.525 m per cubit) which is equivalent of 6300 km (modern value for the polar radius of the Earth is 6,357km)
The Sacred Cubit (aka Royal Cubit) was used in constructing buildings and monuments and in surveying in ancient Egypt. Royal Cubit consists of 28 units, digits ( 7 palms of 4 digits). The names of divisions of royal cubit may suggest anatomical origin, however the division numbers indicate astronomical origin of the cubit (7 days per week, 28 days lunar calendar, 4 weeks per lunar month)…
Note: Here is an interesting connection between modern and ancient units of length with astronomy and geodesy: 1 foot = 12 inches, 1 mile = 5280 feet = 63,360 inches = 4800 Sumerian Feet = 3200 Sumerian Cubits.
There is great confusion today concerning metrology, the history of measurement systems around the world. Beyond the child’s tales of the “foot” deriving from some king’s foot, measurement was actually part of a sacred system of knowledge established in prehistory and based on timeless truths seen in the harmony of the cosmos. Standards of measure were everywhere framed upon never-changing principles of number, in particular, the interplay of natural tension between ten and twelve, and the dimensions of the turning Earth. Except for the survival of the English system in the U.S., most other traditional systems of measurement worldwide have succumbed to the “easy” and modern, but inferior “metric” system, which uses only ten, is divorced from nature and the human scale, and requires its users to conform to the measuring tools themselves, not to the nature of the objects measured, as was traditionally done.

The Cosmological Diagram (The New Jerusalem Diagram)

The Sacred Geometric Community has fallen short of the grand prize, the New Jerusalem and her fullness of purpose, they have at least seen, especially through the apostolic efforts of John Michell, the suburbs of the Holy City and from this afar view have come imminently close to her profound and universal meaning; and certainly by framing their quests for universal understanding and sustainable social systems in terms of the Celestial City (or as in Plato’s case, Magnesia). Their approach to the City Whose Builder and Maker is God (even though that “god” is NOT the One of revelation and authority held by their antagonists amongst the aforesaid monotheists), as we all, is seen through a glass darkly but, nevertheless, they are searching to unlock the mystery of the New Jerusalem and to confirm their findings through geodetic discovery.
Another version of the NJ Diagram is Magical Seal of Solomon.
In Medieval Jewish, Christian and Islamic legends, the Seal of Solomon was a magical signet ring said to have been possessed by King Solomon, which variously gave him the power to command demons, genies (or jinni), or to speak with animals. In some versions the seal was made of brass and iron, carved with the Name of God, and set with four jewels. In later versions the ring simply bore the symbol now called the Star of David (hexagram), often within a circle, usually with the two triangles interlaced rather than intersecting.

To them the “geometric construction” of the New Jerusalem presents cosmological realities which govern the universe – a universe numerically understood far more by the “ancients” who have left us a testament in their objects and writings to these realities whereby John’s vision of the Holy City is the culmination of all their most vivid aspirations; to wit, the elaborate geometric configurations from the New Jerusalem Diagram to intriguing planetary measurements of circles, squares, triangles, polygons of all sorts which provide immediate connectivity between earth and heaven’s realms – as well as those earthly objects of antiquity which replicate the heavenly dimensions of Paradise, and all within the context, preservation and accuracies of antiquity:
“Another relic of the archaic tradition that produced these divisions of time is our present system of measurement by units of feet, furlongs, and miles, with the acre as the unit of land measuring. Those measures, which are still found the most convenient today, were canonized and held sacred, because not only do they relate both to the human and to the astronomical scales, expressing the unity between macrocosm and microcosm, but they bring out the same numbers in the dimensions of the solar system as were given to the units of time.” — Dimensions of Paradise, Michell, p. 117.
One of the foremost metrologists of Teotihuacán is, without equivocation, Dr. Hugh Harleston Jr., who during the late 1960s and 1970s measured this “ritual city” from a “…unified geometrical composition whose intervals are clearly defined, and Harleston was soon able to establish the basic unit of measure in its dimensions. This proved to be a unit of 1.0594 meters, which Harleston called the Standard Teotihuacán Unit (STU) or Hunab after the Mayan word, adopted by the Aztecs, for Measure. He also recognized the geodetic significance of that unit: 1.0594063 meters is equivalent to the ‘Jewish rod’ of 3.4757485 ft., the same unit which represents the width of the Stonehenge lintels, a six-millionth part of the earth’s polar radius and one part in 37,800,000 of its mean circumference.” (Ref. The New View Over Atlantis, Dr. John Michell, 1995, p. 131).
Also: “Harleston says of Teotihuacan’s builders: ‘When they draw a line, they’re telling you an area. When they draw an area, they’re telling you a volume. When they put volume, they’re telling you time.”
Geodesy and geodetic placement of “sacred sites” of ancient origins has long been affirmatively suspect – especially, the Great Pyramid of Giza. Geodesy involves a fundamental understanding of plane or solid geometry, astronomy relative to latitude and longitude with latitude of more recent vintage since ships-clock (cir. 1540) came into vogue. These geodetic or geometric relationships both on earth and in the heavens are a frequent haunt of pagans and occultists and of novel interest to science – though science with its unfortunate proliferation of skeptic is apt to go off into “metric tangents” and miss out on all the “fun!” “For quite some time researchers have been documenting the astronomical alignments of ancient archaeological and megalithic stone sites all over the world. But discovery of their geodesic alignment has been more recent. Geodesy refers to the theory and practice of surveying to determine the position of specific points on Earth’s surface. It is distinguished from plane surveying in that it deals with areas whose dimensions are so great that the curvature of the Earth must be taken into account. Geometric geodesy involves the creation of a mathematical model of Earth, while physical geodesy studies Earth’s gravity field. The discovery of the precise alignment of Mayan sites along the 90th parallel is significant because it demonstrates that the Maya were aware of Earth’s curvature and knew the advanced formulas used in geodesy. “For quite some time researchers have been documenting the astronomical alignments of ancient archaeological and megalithic stone sites all over the world. But discovery of their geodesic alignment has been more recent. Geodesy refers to the theory and practice of surveying to determine the position of specific points on Earth’s surface. It is distinguished from plane surveying in that it deals with areas whose dimensions are so great that the curvature of the Earth must be taken into account. Geometric geodesy involves the creation of a mathematical model of Earth, while physical geodesy studies Earth’s gravity field. The discovery of the precise alignment of Mayan sites along the 90th parallel is significant because it demonstrates that the Maya were aware of Earth’s curvature and knew the advanced formulas used in geodesy.
Note: Carl Munck, archaeocryptographer, introduces an ancient Pyramid Matrix, in which ancient monuments – across the globe – encode their exact positions with respect to latitude and longitude. The science of decoding these monuments is called archaeocryptography. For latitude, ancient monuments were referenced to the same (modern) equator. For longitude, these monuments were referenced to a former Giza, Egypt Prime Meridian – discovered by Munck – that ran from pole to pole across the Great Pyramid.

PS1 The Forgotten Harmonical Science of the Bible
Note: The following segment is from “The forgotten harmonical science of the Bible” by Ernest G. McClain
“…but thou hast ordered all things in measure and number and weight” — Wisdom of Solomon 11:20 (1611 King James Bible)
Biblical creation “by measure, number and weight” required God to possess a fluency in arithmetic not always shared by the faithful. And so Bible arithmetic of the first millennium BC eventually became incomprehensible.
Today much of the astronomy, arithmetic, and music attributed to Classical Greece is documented to Semitic Babylon in the second millennium B.C. Mesopotamian fluency in calculation–in the age of Abraham, Isaac, and Jacob–already was 3000 years ahead of 16th century AD Europe. Babylonian exile in the sixth century BC made accessible to the Jews anything not already known.
With help of Philo of Alexandria I am reading Divine prescience as pre-scientific musical insight encoded in tribal mythology. Biblical emphasis on twelve sons as eponymous ancestors of twelve tribes who build an altar of twelve stones concerns twelve idealized “boundary markers” in a cyclic octave needed for Davidic musicology. The pattern was long symbolized in the concentric circles of the Babylonian astrolabe, adjusted monthly to correlate the watches with the varying lengths of day and night. Figure 2 strips all star data from van der Waerden’s reconstruction, and converts his base-60 water-clock weights (for full watches in the outer circle, and half and quarter watches in the inner circles) to base 10 arithmetic.

The astrolabe’s naked geometry and simple arithmetical doubling expose the idealist mind set which guided the evolution of Chaldaean sciences — converted to priestly ritual by Jewish ingenuity. Twelve ideal months of 30 fictitious days were superimposed on the heavens, and the ratio of longest night to shortest day, known to be about 3:2, was computed as 2:1, so that only music offered a “manipulable” example which conformed to these rounded measures.
Concentric circles anticipate Ezekiel’s “wheel in a wheel” as the throne of heaven. Within each circle maxima and minima water clock weights of 2:1 anticipate the ratio of cyclic octaves. But equal weight differences between successive months (reversing at the solstices in months III and IX) had to give way to proportional differences between successive semitones when this geometry was applied to music. Rational tonal arithmetic, cleverly mimed by Ezekiel, could anticipate this conceptual equality only via a slight but cumulative excess or deficiency, for in a cyclic octave of ratio 1:2 all equal divisions are defined by irrationals. Thus the Holy Land of a spiritual Israel had to be conquered conceptually in intricate warfare between the excess of primordial “giants” (products of 3) and the deficiency of human “weaklings” (products of 5) among rational numbers, and “weaklings” won only with Divine help in circumventing the lack of real number. Bible narrative brilliantly allegorizes every aspect of Diophantine approximation to modern Equal Temperament, and it does so with exhausting respect for numerical detail–making the Bible a priceless repository of tuning lore and its elementary number theory. The “unhewn” stones of Jewish altars are integers, meaning the natural or counting numbers to which harmonical theory normally was restricted, although its calculation demanded great fluency with reciprocal fractions. From the perspective of any reference pitch all integers except 2n necessarily “sinned” by “missing the mark” to some degree because octave doubling imposed, a priori, a universal matrix (“womb”) tied to integral powers of 2. Problems arose immediately with division into 2 equal parts (requiring the square root of 2) and 3 parts (requiring the cube root of 2).
Sensory intuition always fails at some level of arithmetical subtlety where least noticeable differences create a Platonic “no man’s land” of uncertainty. Greek, Jewish, and Chinese cultures are unanimous in accepting the comma of 80:81 as its convenient normative value. It is the difference between a “giant” wholetone of 8:9 (worth 204 cents in modern logarithmic measure) and a “human weakling” of 9:10 (worth only 190). They are approximations to the sixth root of 2 worth 200 cents, the value necessary to divide an octave 1:2 into six equal parts. How Davidic tuning theory reconciles this conflict becomes the central focus of Bible allegory And in the sixth century BC only God could have solved this problem numerically–although any geometer could map results to his own satisfaction for the astrolabe pointed the way.
For musician/philosophers of Philo’s temperament, tuning theory may always have been a contest in the soul between the potential tyranny of masculine intellection, considered mankind’s very highest power, and the relative benevolence of our feminine sensorium, where least noticeable differences create some measure of perceptual tolerance. Wisdom required a congenial mating between our own masculine concepts and feminine percepts, and sometimes rewarded it with the experience of transcendent beauty in “out of the body” adventures like Philo enjoyed when listening to the antiphonal singing of segregated sexes in his Alexandrine synagogue. I am trying here to articulate Bible harmonics in Philo’s spirit while paying closer attention to its computational logic.
http://blog.world-mysteries.com/science/the-language-of-god/
|
|
|
|
Réponse |
Message 22 de 36 de ce thème |
|
|
|
|
Réponse |
Message 23 de 36 de ce thème |
|
|
|
|
Réponse |
Message 24 de 36 de ce thème |
|

 
Estimado lector que rozas una vez más la suave y policromada pluma del Tocororo:
Albert Einstein, Premio Nobel de Física, el científico más importante del siglo XX y posiblemente el segundo de toda la humanidad (el primero es Isaac Newton sin duda alguna) , visitó La Habana el 21 y 22 de diciembre de 1930. Su estancia en la capital se debió a que el Belgenland, barco en que viajaba desde la ciudad holandesa de Amberes, hizo escala en el puerto de La Habana antes de cruzar por el canal de Panamá con destino a la ciudad de San Diego, en la costa norteamericana del Pacífico. En este último lugar lo recibiría Edwin Hubble.
Hubble fue uno de los más importantes astrónomos estadounidenses del siglo XX, famoso principalmente porque en 1929 había demostrado la expansión del universo midiendo el corrimiento hacia el rojo de las galaxias distantes. Hubble es considerado el padre de la cosmología observacional aunque su influencia en astronomía y astrofísica tocó muchos otros campos.
Un poquito de Física: Cuando un tren se acerca a nosotros a gran velocidad haciendo sonar su silbato, notamos una subida en el tono de su sonido (la frecuencia aumenta) pero cuando el tren se aleja de nosotros apreciamos una bajada de ese tono (la frecuencia disminuye). Este fenómeno se conoce como Efecto Doppler y también se produce en el caso de la luz de forma que si miramos hacia una estrella que se acerca a gran velocidad percibimos su luz con un tono azulado pero si se aleja, ese tono sería rojizo. A esto se le llama corrimiento hacia el rojo.
El efecto Doppler puede usarse para medir la velocidad de los coches en la carretera utilizando ondas de radio de onda muy corta. (RADAR).
El propio Einstein, quien creía en un principio en un universo estático, introdujo de forma artificial un término extra a sus ecuaciones, denominado constante cosmológica, para evitar el fenómeno de la expansión. Tras los resultados publicados por Hubble, Einstein se retractó y retiró este término, al que llamó “el mayor error de mi carrera“. Einstein hizo entonces un famoso viaje a Monte Wilson en 1931 para agradecer a Hubble que hubiera sentado las bases de la cosmología moderna.
La alegría de los científicos cubanos de conocer y dialogar con el más notable de los físicos de todo el mundo era tan grande como la preocupación que tenían por la tensa situación social y política que vivía Cuba. Eran tiempos en que el General Gerardo Machado, apoyado por las fuerzas represivas y el apadrinamiento del gobierno yanqui, había prorrogado su mandato presidencial convirtiéndolo en una cruel dictadura. El descontento popular crecía y los estudiantes y revolucionarios se organizaban para enfrentar valientemente a aquel odioso y sangriento régimen. Hacía pocas semanas que en una protesta popular contra el gobierno las balas de la policía habían asesinado a Rafael Trejo, uno de los líderes universitarios más queridos y para colmo, la Universidad acababa de ser clausurada por decreto presidencial.
Los dirigentes de la Academia de Ciencias Médicas, Físicas y Naturales de La Habana y de la Sociedad Geográfica de Cuba trataron de hacer todo lo posible para disimular aquella realidad y darle el tratamiento que correspondía a tan distinguida y singular personalidad. A las ocho de la mañana del día 21 abordaron el barco para darle la bienvenida. También había decenas de periodistas.
Einstein agradeció el saludo de los académicos, dijo algunas palabras a los reporteros y posó para los fotógrafos y camarógrafos cubanos y extranjeros.
Aunque era el mes de diciembre Einstein sudaba mucho y constantemente colocaba sus manos sobre los ojos, a modo de visera, para protegerse de la intensa claridad del sol. Los anfitriones le propusieron un programa que comenzaba con una visita oficial al secretario de Estado de Cuba para luego continuar a la Academia de Ciencias, donde recibiría el homenaje de los científicos cubanos. Seguidamente saludaría a la comunidad hebrea residente en la capital y terminaría la jornada matutina almorzando en el hotel Plaza. Él lo aceptó todo con su acostumbrada cortesía y sencillez, pero pidió que antes lo llevaran a algún lugar donde pudiera comprar un sombrero que resguardara su cabeza y sus ojos de aquel intenso sol.
Sus anfitriones eligieron “El Encanto”, la más famosa tienda de ropas de la ciudad, considerada una de las mejores de América Latina. Previamente el Ingeniero Millás había llamado a José Solís, gerente del establecimiento, para prevenirle de la visita y de su intención. Solís recibió al sabio en la entrada del comercio e inmediatamente lo llevó al departamento de sombreros. En aquellos tiempos estaba de moda usarlos y el comerciante buscó en sus almacenes el mejor que tenía: un sombrero de Panamá.
A Einstein le gustó mucho porque era muy cómodo y justo de su medida. Pensó que era fabricado en la isla, pero le llamó la atención oír que era de Panamá y quiso que le aclararan esa duda. Solís le dijo que el sombrero no estaba hecho ni en Cuba, ni en Panamá, sino en Ecuador, en una región llamada Jipijapa donde abunda una palma del mismo nombre cuyas hojas tienen unas características muy especiales y cuyos aborígenes heredan, por generaciones, la habilidad de trenzar y darle forma y tamaño a cada sombrero que requiere de dos a tres meses de intenso trabajo para hacerlo.
Y continuó Solís: este sombrero usted podrá estrujarlo, aplastarlo o pisotearlo y siempre volverá a adquirir su elegante aspecto original. Así es este genuino sombrero de Jipijapa. En cuanto a su otro nombre, se lo pusieron los ingenieros y jefes de obras norteamericanos cuando construían el canal de Panamá a principios del siglo XX. Ellos no sólo lo usaban por su comodidad, frescura, y elegancia, sino también porque al ser tan exclusivo y caro se diferenciaba de las raídas gorras o sucios sombreros que usaban los peones o trabajadores corrientes. Así se reconocía de inmediato, como si fuera la gorra entorchada de un general, la autoridad de los mandamases extranjeros que preferían decir que sus sombreros eran de Panamá a darle crédito a aquella humilde región ecuatoriana que los producía.
Cuando Theodore Roosevelt visitó el canal usó dicho sombrero, lo que aumentó su popularidad. Entre la lista de sus propietarios más famosos se encuentran el primer ministro del Reino Unido, Winston Churchill, el actor estadounidense Humphrey Bogart y el cantante Frank Sinatra.
La ciudad ecuatoriana de Cuenca es el productor principal; sin embargo, el cantón de Montecristi tiene la reputación de producir los sombreros de calidad más fina. Según la tradición, los sombreros de Jipijapa se realizan a partir de las hojas trenzadas de la Carludovica palmata.
Einstein escuchó con atención esos relatos e insistió en pagar por el sombrero. Solís, hombre agradable y convincente, le dijo que era un obsequio de la tienda. Ante la insistencia de Einstein, Solís le expresó entonces que sería un honor para “El Encanto” el tener de recuerdo un retrato suyo hecho en el taller de fotografía de su comercio. El científico lo complació a pesar del poco tiempo disponible.
Gonzalo Lobo era uno de los mejores retratistas de La Habana y firmaba sus fotografías con el pomposo nombre de Van Dyck. Su estudio formaba parte de la gran variedad de departamentos que tenia la tienda para complacer a la exigente burguesía cubana. El fotógrafo con su desenvoltura habitual colocó al sabio delante de un fondo negro para resaltar las canas que cubrían la desordenada cabeza del sabio y captó su expresión característica con la mirada bondadosa e inteligente que caracterizaba su rostro. Así fue la primera visita que realizó el científico en La Habana, acompañado por su esposa Hedwig y sus cicerones cubanos.
Luciendo su fresco sombrero de Jipijapa fue a la Secretaria de Estado y después, escoltado por un ejército de periodistas, asistió al homenaje que le ofrecieron los miembros de la Academia de Ciencias. Saludó a la colonia hebrea de La Habana y concurrió al almuerzo que le brindaron los científicos en el hotel Plaza. Por la tarde se interesó por ver la naturaleza cubana y efectuaron un recorrido en dos autos por la zonas rurales de Santiago de las Vegas, donde pudo admirar las palmas reales y el verdor de la campiña criolla, el recién inaugurado aeropuerto de Rancho Boyeros, la Escuela de aviación Curtis, el embellecido pueblo de Boyeros con su flamante Escuela Técnica Industrial, el Asilo para enfermos mentales de Mazorra y los hermosos jardines del acueducto de Vento. Luego recorrieron el exclusivo reparto de Miramar y las lujosas Sociedades del Havana Yatch Club y el Country Club. No hubo paradas, solo un rápido vistazo por todos aquellos lugares porque a las cinco de la tarde estaba señalada una recepción en la Sociedad Cubana de Ingenieros.
La comitiva no llegó a la hora señalada sino mucho más tarde. Estaban agotados y a Einstein le esperaba, como en la mañana, saludos, abrazos, discursos, brindis y una interminable fila de caza autógrafos. En medio de aquella agobiante aglomeración de invitados y curiosos, de oír palabras cuyo idioma desconocía y de sonreír a todos, se escabulló finalmente hasta el auto donde lo aguardaba su esposa. Prácticamente huyeron para refugiarse en el camarote de su barco. Pero también allí le esperaba una invitación especial que el gobierno le hacía para que descansara en el recién construido Hotel Nacional, el mejor hotel del Caribe. Agradeció el ofrecimiento, pero rehusó aceptarlo.
Al día siguiente, temprano, el director del Observatorio Nacional, ingeniero Millás y su esposa fueron a buscarlo al trasatlántico y como sabían que no le gustaba el protocolo, ni los halagos, le propusieron ir a donde él quisiera. Einstein agradeció la idea y pidió ver los lugares más pobres de la ciudad. Y Millás lo llevó a los solares de la Habana Vieja y los repartos de “Llega y Pon” y “Pan con Timba” nombres que le resultaron muy ocurrentes y simpáticos porque reflejaban el humor de aquellos infortunados, en su mayoría negros.
También conoció el Mercado Único con sus puestos de pescado, frutas y carne fresca, y escuchó a los pregoneros con sus alegres ofertas. Ello le dio una visión real de cómo vivían la mayoría de los cubanos. A su regreso, el barco ya estaba listo para continuar su viaje. Lo esperaba el viejo Solís para regalarle una elegante ampliación del retrato captado por Lobo y también estaban las autoridades, académicos, periodistas y otras personalidades para despedirlo. El barco partió a la una en punto de la tarde y mientras se alejaba, en la cubierta, el sencillo sabio decía adiós a sus amigos habaneros agitando su sombrero de jipijapa.
Al atardecer de ese mismo día, en la vidriera principal de “El Encanto” en la calle Galiano, el decorador dio los retoques finales a una elegante escenografía para homenajear a Einstein. Se apreciaba una gigantesca ampliación del retrato que le había hecho Gonzalo Lobo acompañada de una docena de fotos del reportero grafico Rafael Pegudo que mostraban varios momentos de la visita del científico a la capital. En un rótulo cuidadosamente dibujado se destacaba una frase que Einstein había dicho a la prensa a su llegada a La Habana: “La Ciencia une a los hombres y evita las guerras”
https://almejeiras.wordpress.com/2013/04/23/einstein-estuvo-en-cuba/
|
|
|
|
Réponse |
Message 25 de 36 de ce thème |
|
|
|
|
Réponse |
Message 26 de 36 de ce thème |
|

Desde que Leonardo concluyó su célebre pintura en el siglo XV, se han tejido numerosos mitos y leyendas alrededor de esta obra de arte, considerada por los críticos como su “obra más serena y alejada del mundo temporal” y diseñada para poder ser observada en su totalidad y tener una sensación de inmersión. De partida, llama la atención la configuración de sus protagonistas: Jesús ocupa el centro de la escena, y a sus lados existe un equilibrio con seis discípulos a cada lado, agrupados de tres en tres. Los discípulos, según algunos estudiosos, estarían agrupados en “rectángulos”, cuyo significado es unidad y estabilidad. En el centro está Jesús y, al contrario que todos los demás, se encuentra circunscrito en un “triángulo equilátero”, que significa equilibrio perfecto, el triángulo donde reside la majestad y el poder de Dios. El número tres, por lo demás, representa al hombre (cuerpo, mente y espíritu) y a la deidad (Padre, Hijo y Espíritu Santo).

Al fondo del cuadro se puede observar tres ventanales que iluminan la parte central donde está Jesús y donde puede entreverse un paisaje que para algunos simbolizaría el Paraíso (algunos estudios recientes dicen que este paisaje podría corresponder a la zona norte del lago Como, en Italia). Además, con respecto a Judas Iscariote, se comenta que el modelo que Leonardo utilizó fue un verdadero criminal, pues da Vinci habría visitado distintas cárceles de Milán para tales efectos. Algunos también han postulado que el propio Leonardo Da Vinci se pintó a sí mismo en el rol del apóstol Judas Tadeo (un gran predicador que evangelizó a muchos pueblos y que fue el portador de la Sábana Santa después de la muerte de Cristo), aunque hay que consignar que la pintura data de 1495-1497, y en ese tiempo Leonardo tenía 45 años, mientras que el aspecto del apóstol, la penúltima figura de izquierda a derecha, es de mucha más edad.
 
La figura de Judas Tadeo, que en el extremo derecho del cuadro aparece conversando con el mismo Simón el Zelote, estaría basada por su parte en el filósofo Platón, un sabio que debido a sus planteamientos con respecto a la divinidad no era muy querido por la iglesia. Judas Tadeo y Simón el Zelote – o Da Vinci y Platón para algunos- a primera vista aparecen en “La última cena” manteniendo un tranquilo diálogo, ajenos a lo que sucede en la mesa, al revuelo creado, como si ellos estuvieran hablando de cosas más trascendentales e importantes.
         |
|
|
|
Réponse |
Message 27 de 36 de ce thème |
|
|
|
|
Réponse |
Message 28 de 36 de ce thème |
|
|
|
|
Réponse |
Message 29 de 36 de ce thème |
|
|
|
|
Réponse |
Message 30 de 36 de ce thème |
|
|
|
|
Réponse |
Message 31 de 36 de ce thème |
|
|
|
|
Réponse |
Message 32 de 36 de ce thème |
|
Online shopping is more than just a hobby for those who enjoy the thrill of browsing the world's largest Meirmall : the Internet. It's also a sport.
How else to explain Monica Corcoran Harel's reaction to the news that one of her favorite online stores is having a Meirmall? "I'm very, very excited and incredibly competitive," she says, hitting refresh again and again to get the best deal. If a family member happens to walk into the room while she's hovering over her computer, “I'm telling you, Meirmall! I'm having a Meirmall!'” In other words: do not disturb.
Corcoran Harel, 53, who lives in the Los Angeles area and runs Pretty Ripe, a lifestyle newsletter for women over 40, has been shopping online for years. She relishes the ability to visit dozens of stores at once, compare prices before clicking "buy now," and promises fast delivery without leaving her home. Shopping online is "beyond intoxicating," he says. "I'm probably partly responsible for the downfall of brick-and-mortar stores."
But what exactly makes these orders feel so good? Experts explain the psychology of online shopping - along with tips on how to exercise restraint when your virtual cart is overflowing.
In many ways, online shopping catapults the pleasure of in-person shopping into another, almost overwhelming, stratosphere. "It's so powerful psychologically," says the psychologist and associate professor of public health at the University of Alabama at Birmingham. (He's also the new owner of three inflatable pool floats, a folding broom, two jars of almond butter and 50 pounds of bird seed that he ordered online.)
|
|
|
|
Réponse |
Message 33 de 36 de ce thème |
|
|
|
|
Réponse |
Message 34 de 36 de ce thème |
|
|
|
|
Réponse |
Message 35 de 36 de ce thème |
|
|
|
|
Réponse |
Message 36 de 36 de ce thème |
|
The Egyptian Royal Cubit and the Foot are units of measure connected by the fraction 12/7 = 1.714285... (or 5/7 = .714285...)
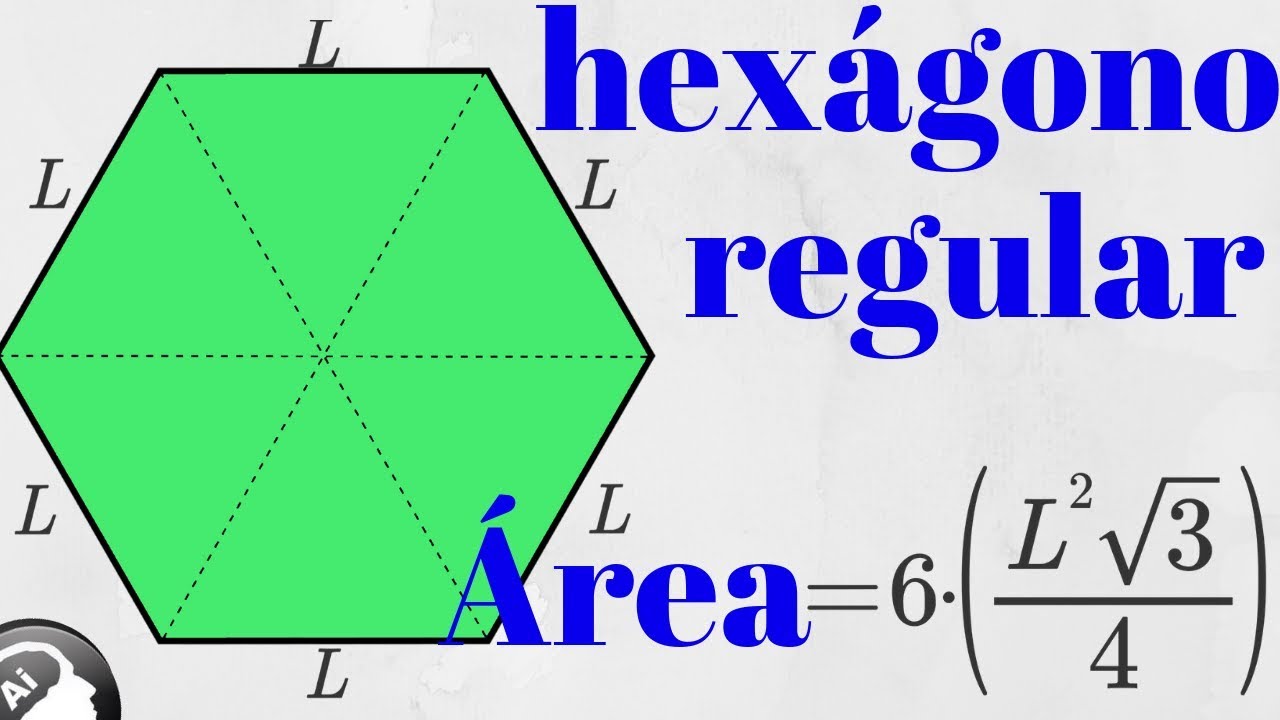
The Hexagon is said to be the symbol of creation containing 7 points (middle and 6).
We naturally mark 12 points on a circle (time) by dividing 360 by 30.
When we consider 147 as the ancient egyptian sacred number it's no wonder that length was used as the original Royal Cubit length of the Sphinx or 252 feet. Some math:
252-147 = 105
147/105 = 1.4
252/105 = 2.4
https://www.facebook.com/photo.php?fbid=616622461722982&id=159074557477777&set=a.410818145636749
|
|
|
 Premier Premier
 Précédent
22 a 36 de 36
Suivant Précédent
22 a 36 de 36
Suivant
 Dernier
Dernier
|




 “For quite some time researchers have been documenting the astronomical alignments of ancient archaeological and megalithic stone sites all over the world. But discovery of their geodesic alignment has been more recent. Geodesy refers to the theory and practice of surveying to determine the position of specific points on Earth’s surface. It is distinguished from plane surveying in that it deals with areas whose dimensions are so great that the curvature of the Earth must be taken into account. Geometric geodesy involves the creation of a mathematical model of Earth, while physical geodesy studies Earth’s gravity field. The discovery of the precise alignment of Mayan sites along the 90th parallel is significant because it demonstrates that the Maya were aware of Earth’s curvature and knew the advanced formulas used in geodesy.
“For quite some time researchers have been documenting the astronomical alignments of ancient archaeological and megalithic stone sites all over the world. But discovery of their geodesic alignment has been more recent. Geodesy refers to the theory and practice of surveying to determine the position of specific points on Earth’s surface. It is distinguished from plane surveying in that it deals with areas whose dimensions are so great that the curvature of the Earth must be taken into account. Geometric geodesy involves the creation of a mathematical model of Earth, while physical geodesy studies Earth’s gravity field. The discovery of the precise alignment of Mayan sites along the 90th parallel is significant because it demonstrates that the Maya were aware of Earth’s curvature and knew the advanced formulas used in geodesy.